What Changes Should Be Made for the Next Edition of the Common Core Standards?
This article presentation was given by UCSMP Director Zalman Usiskin at the annual meeting of the National Council of Teachers of Mathematics in New Orleans,LA, on April 10, 2014.
In November 1992 at the University of Chicago and at the annual meeting of the National Council of Teachers of Mathematics (NCTM) in 1993, I gave a talk titled “What Changes Need to Be Made for the Second Edition of the NCTM Standards?”. My first motivation for these talks was obvious – I felt that some changes were needed. But my second and equally important motivation was that no one was talking about when a major rethinking of the NCTM Curriculum and Evaluation Standards for School Mathematics (1989) would be appropriate, and I felt that maybe a talk on the subject would help get the ball rolling. I think it had some effect, leading to NCTM’s Principles and Standards for School Mathematics (2000). That same motivation drives me here. I think changes are needed in the Common Core , but no one is talking about when we are going to rethink the Common Core and I think it is not too early to get the ball rolling.
Not everyone is on the Common Core bandwagon. That would be too much to expect. A movement as large as this one will always draw opposition. The loudest single voice has come from Diane Ravitch (2014) but there are many other voices. There is organized opposition on general grounds that cut across both language arts and mathematics in almost every state that has adopted the Common Core. Some people are opposed because of the content of the Common Core, some because of the loss of local control, some because of the lack of flexibility, some because of the testing, some because of the cost in time and money, and some because they foresee huge numbers of students failing the Common Core tests, being held back, and leaving school whereas without the tests they would remain in school.
In both language arts and mathematics, it’s not the content of the Common Core that has caused the most opposition; it’s the tests. As the slide indicates, a number of states have withdrawn from one or both of the test consortia, and two weeks ago [24 Mar 2014] Indiana formally withdrew from the Common Core entirely, though its mathematics standards remain virtually the same as in the Common Core.
Table 1: States that have withdrawn from one of the two CCSSM test consortia
| State | Withdrawn From |
|---|---|
| Alabama | PARCC & Smarter Balanced |
| Florida | PARCC |
| Georgia | PARCC |
| Indiana* | PARCC |
| Kansas | Smarter Balanced |
| Kentucky | PARCC |
| Oklahoma | PARCC & Smarter Balanced |
| Utah | Smarter Balanced |
*Indiana has divorced itself from the Common Core entirely. Alaska, Minnesota, Nebraska, Texas, and Virginia never joined the Common Core; Pennsylvania has only been an advisory member of both consortia.
As best as I can determine, there are now (April 2014) 14 PARCC states plus the District of Columbia and 22 Smarter Balanced states. My purpose here is not to discuss the various sides on this issue but these withdrawals make it clear that there is major opposition to the Common Core. And when the more vocal criticism comes once the testing begins, it will be useful to have thought already about what changes should be made.
The Current Scene and What Got Us Here
To understand the process of doing a revision of the CCSSM standards, it helps to understand what led to the CCSSM.
The first event that can be said to have led to the CCSSM was the appearance of the NCTM Curriculum and Evaluation Standards of 1989. These standards set a precedent for national standards in a subject matter field, and their authenticity for setting national goals was established when NSF decided in the early 1990s to fund a variety of curriculum development projects at elementary, middle, and high school levels to explore ways of implementing these standards. This gave the first purpose of standards: to set goals for the curriculum. And this is a first purpose of the CCSSM.
The second event leading to the Common Core was the appearance of A Splintered Vision (Schmidt, McKnight, and Raizen, 1996) as part of the Third International Mathematics and Science Study, TIMSS. The authors of this document argued that a major reason our mean score was behind other nations on TIMSS was due to the lack of a consistent vision across the nation. This gave the second and third purposes of the CCSSM: to bring the U.S. score on international assessments up to that of the highest countries of the world, and to do this by having a common curriculum for all students in the country and high-stakes testing to ensure that the curriculum gets to the students.
The third event, at about the same time, was the entry of some mathematicians expressing the view that a major reason for low scores in California and other states on National Assessment was the NCTM Standards and the curricula based on them. (Never mind that those curricula were not yet being used.) These mathematicians saw greater numbers of students taking what are called remedial mathematics courses in college and greater numbers of foreign students in their graduate courses and no greater numbers of students majoring in mathematics despite the assertions of so many that mathematics is as important as ever in our technological age. They argued against what they called the “fuzzy math” of the NCTM Standards and the constructivism that was fostered in the NCTM Professional Teaching Standards that had appeared in 1991. This gave two more reasons for the CCSSM: to eliminate the impact of the NCTM Standards and the NSF curricula based on them, and to replace that curriculum with one designed to prepare mathematics majors.
Fourth was the No Child Left Behind legislation of 2001 that established the U.S. Department of Education’s entry into the scene, set annual testing of schools and penalties for low-performing schools. This gave us two more reasons for the CCSSM: to close the achievement gap between Blacks and Whites and between Hispanics and Whites and to improve teaching by using test scores to get rid of low-performing teachers.
Finally, the CCSSM were pitched to states during a time of a recession, when states were looking for ways to reduce their budgets, and states hoped that by joining together to create standards and assessments of those standards, better results could be obtained for less money. The logic is compelling: get the best people in the nation in a room, give them the task, and the result will be better and the process less costly than if 50 groups of people get together in 50 rooms for the same reason.
What Changes Should Be Made in the Process of Creating Standards?
With this background, we can talk about the process of creating a next edition of standards.
The need for someone in charge
A Splintered Vision begins with the following statement:
“There is no one at the helm of U.S. mathematics and science education. In truth, there is no one helm. No single coherent vision of how to educate today’s children dominates U.S. educational practice in either science or mathematics. There is no single, commonly accepted place to turn to for such visions. The visions that shape U.S. mathematics education are splintered. This is seen in what is planned to be taught, what is in textbooks, and what teachers teach.” (p. 3)
The thesis of this argument of Bill Schmidt, Curtis McKnight, and Senta Raizen is that a major reason our mean score was behind other nations on TIMSS was due to the lack of a consistent vision across the nation.
In this regard, in the last school year I heard Phil Daro, one of the three writers of the Common Core, say that there is no one in charge of the Common Core. The work group appointed to develop the Common Core committee no longer exists. The three authors, he, Bill McCallum, and Jason Zimba, have no official capacity. There is no organization in charge, not the National Governors Association, not the Council of Chief State School Officers, not Achieve, not NCTM nor any other mathematics education organization. No change can be made at present in the Common Core because no person or organization has the responsibility. Phil said, it is “not clear who the next people will be, not even clear who will organize it.”
The problem with having no one responsible for the Common Core is that no one is accountable. So we have a situation in which every student, teacher, and public school in grades 3-11 in many states is being held accountable for mathematics performance under guidelines for whose preparation no one is accountable. You might argue that the individual states are accountable, and to a degree they are accountable if they made changes in the Common Core, but it is hard to call them accountable if, like my own state Illinois they merely have accepted the Common Core as is. They can simply say: we did not make the rules; we are simply going by the rules. That is not accountability because there is no one to take the blame for the rules.
This must change for any next version, and I believe that we in mathematics education must take back the leadership for school mathematics that has been wrested from us by entities that either have no members or are political rather than educational in nature. The natural organization to take the leadership is NCTM, but it may be appropriate for NCSM to do what it did 37 years ago, when it came out with its Position Paper on Basic Mathematical Skills and jump-started the process of extricating us from the back-to-basics movement. If you agree with me, I hope that you will badger these national organizations to take back the leadership in mathematics education. Write their presidents, which after this meeting will be Diane Briars for NCTM and Valerie Mills for NCSM.
The need for testing new standards
One of the early complaints about the Common Core was that the work group did not have enough time to do its work and gave only about a month at the very end for outside comments on the document, far too late for any significant criticisms to be accommodated. One of the major points made by Diane Ravitch and others is that the Common Core states have adopted a curriculum that has never been tested by anyone anywhere. Indeed, most of these states adopted the standards before they appeared!
So any time line for a second edition of the Common Core should allow enough time for development, public comment, revision, testing of the new ideas, and lead time before implementation.
In Japan, for a number of decades there has been a planned revision of the curriculum every ten years. I use Japan as an example because their system for changing curriculum is the most refined known to me. They call their document the course of study. It takes two or three years for the course of study to be implemented – it is not all implemented in one year. For instance, the current course of study in Japan was made public in March 2008 and was implemented fully in April 2011. (The Japanese school year starts at the beginning of April.) Two years after implementation, when there have been some results of student testing, a committee meets and makes suggestions for the next course of study. This is five years before the next course of study goes into effect. These suggestions are let out for public debate, and then the final document – a revised course of study - appears about two years before its required implementation to give time for teachers to be trained in whatever is new.
The U.S. is not so organized. Some places began teaching the Common Core a year ago, so that the testing in 2014-15 will be the third year of implementation, pretty good, but some places you know will not implement until the year of testing. In general, I think people have been satisfied with the amount of lead time for implementation and training. We will have two years of test results by June 2016. So a committee could begin meeting then and possibly come out with revised standards in a year, give a year for debate and modifications, and then two years for implementation, so that in 2020 there would be a new version of the standards. So I think that the goal should be for revised standards in 2020.
The need for places to test
If we want a change to work on a large scale, we need to try it out at least on a small scale. Not only was this aspect of the Common Core completely neglected, but in the present implementation of the Common Core there is no place for trying out new ideas, because all students in all public schools in the population are being tested. You might argue that we could test in private schools, but those schools are special in that they do not have to accept all students, a luxury not available to public school students, and many if not most of them are accredited by their states and thus under the testing rules of the Common Core. The difficulty of trying new ideas is a huge problem associated with the Common Core. President Obama repeatedly calls for innovation, but the Common Core stifles innovation among our teachers, and where else are our students supposed to learn to innovate if not from their teachers?
Last year, the U.S. Department of Education launched a “2013 Investing in Innovation Competition” (U.S. Department of Education, 2013). There were eight priority categories for innovation, including one titled “Improving STEM education”, but I don’t see how a school could afford to take the risk of adopting a new curriculum if its students are going to be tested on the Common Core.
Japan has a built-in mechanism for trying out its new ideas – its schools that are affiliated with its teacher-training universities. In March of last year I was at two of those universities; each has about a dozen affiliated schools. These are the places in which prospective teachers do their student teaching and in which ideas such as lesson study have been honed. We have no such mechanism built into our system. We need to develop a mechanism immediately by which a school can petition to implement a curriculum different from the CCSSM.
The need for knowledgeable writers
Obviously the standards should be written by knowledgeable people. But what knowledge? The people who write the standards should be experts in curriculum, in various ways in which each mathematical idea can be developed, how the development can be modified for different populations and how much time is reasonable for the ideas to be learned and applied. The writers should have experience teaching students at the levels for which they write. Some should be aware of the mathematics curricula of other countries, and many should be aware of the latest developments in curriculum. And they should have experience writing materials for students so that they are aware of the choices, difficulties, and subtleties of organizing and sequencing content for optimal learning.
The creation of standards is a very difficult task. Any revised standards and the accompanying explanatory matter should be written by people who specialize in mathematics curriculum, that is, by our most gifted teachers, supervisors, authors, and professors in mathematics education, not by people in related fields, regardless of how smart they might be. The authors need to be aware of the possible consequences of their decisions, both intended and unintended. This is more justification for the leadership in a next edition being held by NCTM and NCSM.
What Changes Should Be Made in the Substance of the Standards?
We know that the CCSSM were quickly written and let out for only 22 days for public comment. It is to be expected that the CCSSM contain some major errors in judgment.
I cannot give detailed thoughts regarding the 8 Standards for Mathematical Practice and the 385 Standards for Mathematical Content in an hour or even a couple of hours, so I will concentrate on general aspects of the standards themselves that I believe stand out as needing to be rethought.
Connect the SMP with the Mathematical Content Standards
Many mathematics educators stress that the essence of the Common Core lies in the Standards for Mathematical Practice, the SMP. If you have attended sessions at conferences, you have all have heard this often ever since the CCSSM appeared.
But a year ago I heard Bill McCallum speak about this in his role as a panelist on two panels at the joint mathematics meetings. He said he knew that some people thought the Standards for Mathematical Practice were the key things in the Common Core, but he asserted that is not true because the Standards for Mathematical Practice should be practiced regardless of the mathematical content. The essence of the Common Core, he said, was in the laying out of the Content Standards, that is, in the scope, sequence, and timing of those standards, grade by grade.
I agree with Bill’s assessment of the essence of the Common Core, not just because he was one of the writers but because of the relative importance given to the SMP and the Standards for Mathematical Content in the document. The Standards for Mathematical Practice are detailed in 3 pages. In contrast, the Content Standards occupy over 60 pages. We might compare this split with the NCTM Principles and Standards for School Mathematics of 2000. The PSSM devote 152 pages specifically to the Content Areas of Number and Operations, Algebra, Geometry, Measurement, and Data Analysis and Probability, and 147 pages to the Process Standards of Problem Solving, Reasoning and Proof, Communication, Connections, and Representation.
The tension between the two camps – the camp of those who view the SMPs as the key aspect of the Common Core and those who view the Content Standards as the key aspect – is sometimes pushed into the background by those who argue that the key aspect of the Common Core lies not in the word “core” but in the word “common”, that it is more important for the U.S. to have a common curriculum in order to improve mathematics education in the country than to quibble about aspects of that curriculum. But when people say “common”, they are talking about the content standards, not the SMP.
I think a revised standards needs to have a great deal of rhetoric and many examples per grade indicating how the Standards for Mathematical Practice can be instantiated at that grade along with the Content Standards.
Rethink the meaning of “Core”.
Although these new standards are called the “Common Core”, I think most teachers believe this core is larger in virtually every year than the curriculum it is supposed to replace. If the current curriculum is overloaded, what can you say about a curriculum that is even larger? Furthermore, all students are expected to learn the same core, and no country in the world keeps all students in the same curriculum through 11th grade. A revision of the standards needs to rethink the meaning of “core” so that it has practical applicability for all students.
I think I understand the logic of the Common Core writers in this regard. I think we all believe that all students should graduate high school. It is also reasonable to believe that all high school graduates should be prepared for college. However – and this is a big “however” – being prepared for college does not necessarily mean that every student needs to be prepared for college-level mathematics. As a mathematics zealot, I want every person to understand the major ideas of my subject, but that does not mean that everyone has to be able to go in and work with those ideas the same way that a mathematics major might.
Here are some examples: Should all students be expected to compute with paper and pencil with decimals of any length? We can see that this is the intent by comparing the 6th-grade standards with the 5th-grade standards.
Standard 5.NBT: “Perform operations with multi-digit whole numbers and with decimals to hundredths.
5. Fluently multiply multi-digit whole numbers using the standard algorithm.
7. Add, subtract, multiply, and divide decimals to hundredths, using concrete models or drawings and strategies based on place value, properties of operations, and/or the relationship between addition and subtraction; relate the strategy to a written method and explain the reasoning used.” (CCSSM, p. 35, emphasis mine)
Standard 6.NS: “Compute fluently with multi-digit numbers and find common factors and multiples.
2. Fluently divide multi-digit numbers using the standard algorithm.
3. Fluently add, subtract, multiply, and divide multi-digit decimals using the standard algorithm for each operation. (CCSSM, p. 42, emphasis mine)
In the 5th grade standards there is a limit on decimal places that has been somewhat customary for the past few decades. In the 6th grade there is no such limit. Paper-and-pencil algorithms for multiplication and division of decimals are obsolete for most adults, who will use calculators to obtain the answers if and when they ever would need them. And I need not tell anyone in this room that it takes a typical student many months to learn these algorithms.
A high school standard is that students should be able to divide polynomials. There is no way this is appropriate for all students. Again, there exists technology that can do these algebraic calculations with more speed and accuracy than students can. Here the Common Core authors missed the opportunity to bring the mathematics curriculum of the United States into the 21st century.
On the other hand, some of the standards I call STEM standards at the high school level should be for all students. Matrices and vectors arise in many kinds of data situations, they are part of the language of mathematics, and students should at least have seen them. Should all students be taught the mathematics of lotteries? – yes, because they are ubiquitous in today’s culture.
Even out the Expectations Over the Grades
I do not know how the work group and the writers worked to get the grade placements of the standards. One possibility is that they worked up from kindergarten and down from grade 12, because the middle school curriculum is packed. In particular, the content expected to be mastered in Grade 6 is massive. Here is the 6th-grade overview:
Ratios and Proportional Relationships
- Understand ratio concepts and use ratio reasoning to solve problems.
The Number System
- Apply and extend previous understandings of multiplication and division to divide fractions by fractions.
- Compute fluently with multi-digit numbers and find common factors and multiples.
- Apply and extend previous understandings of numbers to the system of rational numbers.
Expressions and Equations
- Apply and extend previous understandings of arithmetic to algebraic expressions.
- Reason about and solve one-variable equations and inequalities.
- Represent and analyze quantitative relationships between dependent and independent variables.
Geometry
- Solve real-world and mathematical problems involving area, surface area, and volume.
Statistics and Probability
- Develop understanding of statistical variability.
- Summarize and describe distributions. (CCSSM, p. 41)
Notice the fundamental ideas: This is the big year for ratio. There is the expectation of computational fluency with decimals of any length, mentioned above. There is the extension of the arithmetic of fractions to deal with rational numbers. There are the beginnings of both algebra and functions. There is the geometry of area and volume. I estimate that there is about 45 weeks work for a typical class to cover this content. But it is not just the time it takes, it is that so many of the ideas are fundamental.
The critical nature of the middle school standards was recognized by the writers, for at the end of the Common Core is the following remark:
“Indeed, some of the highest priority content for college and career readiness comes from Grades 6-8. This body of material includes powerfully useful proficiencies such as applying ratio reasoning in real-world and mathematical problems, computing fluently with positive and negative fractions and decimals, and solving real-world and mathematical problems involving angle measure, area, surface area, and volume.” (CCSSM, p. 84)
But except for computation with negative numbers, this description does not apply to grades 6-8; it all is in grade 6! There is simply too much in this year.
Another aspect of the unevenness of the expectations comes with the simplistic idea that the way to get students to algebra in 8th grade is simply to combine 7th and 8th grades into one year for those students or grades 7 through 9 into two years. This recipe might have worked in the early 1980s, when grade 8 was almost entirely a review of grade 7, but it does not work with current curricula that have higher expectations, and will not work with the common core that has still higher expectations.
Rethink the sequence and timing of the number strand.
The number strand in the Common Core reflects two views, one antiquated and the other I believe to be simply wrong. The antiquated view is that children of the 21st century should learn about numbers in the historical order of their appearance – with the exception of 0. The wrong view is that children should not encounter numbers in class until they can be rather rigorously mathematically constructed. I say these views are reflected in the standards because I am not certain of the extent to which they were consciously applied. Here is what I mean.
Historically, fractions preceded decimals, and in the U.S. curriculum, that was the order of teaching until about 50 years ago. The order changed because children today see decimals everywhere, with money, with times of events in sports, with results of searches on the internet, and so on. They see fractions in far fewer places. Consequently, not only because the algorithms for dealing with decimals follow closely the algorithms for dealing with whole numbers, but also because students have experience with decimals that can be applied to fractions, most modern curricula do decimals simultaneously with fractions. Furthermore, converting fractions to decimals provides substantiation for the fact that different fractions can name the same number. But in the Common Core, operations with fractions precede operations with decimals, and indeed, fractions are used to develop decimals. From a rigorous mathematics standpoint, this is fine, but that is college-level rigor that does not have to be foisted on young children.
Historically, negative numbers appeared late, in the 16th century just before decimals, and mathematicians found them more difficult to accept than decimals. So when I went to school, negative numbers were taught at the beginning of first-year algebra, after the rest of arithmetic. But large numbers of curricula world-wide have shown that students can understand the uses of negative numbers in situations with two opposite directions, they can understand that if you have 2 dollars and buy something that costs 5 dollars, you are 3 dollars short, and this leads to 2 – 5 = -3. So some curricula introduce negative numbers in grade 2. But the Common Core does not want to introduce these numbers until grade 6, reflecting a view that if something came late historically in mathematics, it must be more difficult than something that came early.
Over the years, a few mathematicians have said to me that they don’t like the fact that in many books, real numbers have been described as those numbers that can be written as finite or infinite decimals, because the rigorous development of infinite decimals requires the study of limits typically begun in calculus. Real numbers were not developed rigorously by mathematicians until the 19th century, so these mathematicians feel that if something developed late in mathematics it must be really non-intuitive, and the Common Core mirrors this view by ignoring infinite decimals completely. These mathematicians have it all wrong. We get our intuition about real numbers not from the rigorous definition of a real number using least upper bounds, nested intervals, or Dedekind cuts. We get our intuition from the infinite decimals and the number line, and that comes as early as when the answer to a division problem is a repeating decimal. The Common Core recognizes the power of the number line but does not take it far enough.
Use technology to do mathematics.
Not one paper-and-pencil skill in algebra or arithmetic has been taken out of the curriculum. In fact, since decimals of all lengths and significant algebra skills are required of all students, the breadth of paper-and-pencil skills has increased.
The Common Core writers were clearly worried about the early introduction of calculators. There is not even a single mention of calculators in either the narrative or the specification of content standards for grades K-8 despite the fact that this is a curriculum that supposedly is to prepare students for work in a technological society. The CCSSM ignore the world of today; they are 40 years out of date when it comes to considering how mathematics is encountered and used in today’s world by everyday citizens. The standards speak often of “the standard algorithm” for doing an arithmetic operation. If we define the “standard algorithm” for an operation as the algorithm that is used by educated adults, and in particular if we want fluency – by which is meant speed and accuracy - then the standard algorithms in today’s world for work with multiplication and division of multi-digit whole numbers and decimals involve a calculator, and the most important skills lie in determining whether the answer one gets is reasonable, and in rounding the answer off to an appropriate number of decimal places. It is important to note that Karen Fuson and Sybilla Beckmann (2012/2013), two people who influenced the Common Core, have pointed out that there is no arithmetic operation for which there is just one standard algorithm.
Ironically, the Standards do not seem to follow a line in their own Standard Mathematical Practice regarding technology.
“Proficient students are sufficiently familiar with tools appropriate for their grade or course to make sound decisions about when each of these tools might be helpful, recognizing both the insight to be gained and their limitations.” (CCSSM, p. 7)
How can teachers teach students to make sound decisions about the use of calculators if the students do not have them and so are not sufficiently familiar with them?
Consider including important content missing from the current version.
Despite a core that is much more than a full curriculum in places, I believe there are eight serious broad content areas missing from the core.
- Quantitative literacy; connections with other subjects in the curriculum
We are in an age in which mathematical language and ideas are ubiquitous. Food packages list ingredients and recommended daily allowances to help people make wise decisions regarding what they eat. Financial matters involve more than the simple interest, tax, and discounts that are found in Grade 7 as applications of percent. Dealing with demographic and other data is important for understanding major issues of our day such as global warming, population growth, and government spending. The importance of understanding the mathematics one encounters in everyday matters is described by such terms as numeracy, quantitative literacy, or financial literacy, and the standards wrongly assume that by being fluent with computation and understanding the uses of arithmetic operations, with which they do a very good job, a student would have these understandings. These matters do more than supply applications of mathematics and contexts for learning our subject. They help students understand the value of what they are learning and they can help students develop robust concepts of number and operation. - Mathematics for trades and other careers not requiring a college degree are huge and missing because the committee wrongly felt that the best mathematics for everyone – and I repeat, for everyone – is the mathematics that a student taking college mathematics needs to know.
- Data in grades 1-4
There is almost no attention given to data before grade 5 except a strange obsession with data that comes from measurements of lengths in objects. I wonder why the writers did not adopt standards following the well-known and carefully-written American Statistical Association Guidelines for the Assessment and Instruction of Statistics Education (GAISE) published three years before the Common Core appeared (American Statistical Association, 2007). - Discrete mathematics (prime numbers, logic, algorithms, networks)
The assumption in the Common Core is that the best mathematics for everyone is more narrow than the mathematics needed for college; it is the mathematics needed for calculus and statistics. There is disregard for the discrete mathematics that is fundamental in computer science, even in the extra mathematics for those in high school who will take college mathematics, the standards that I call the STEM standards. - Applications other than probability in the 9-12 STEM standards
The 9-12 STEM standards designed for our best students have a major weakness. There are no applications other than probability in those standards. In fact, as I have mentioned, some of those applications – like lotteries – should be for all students, in the core and not starred. - The metric system (though metric units are mentioned in examples)
It is amazing that the metric system, with its connection to the decimal system, is not part of the core, though metric units are mentioned in some examples. I believe this is another consequence of the archaic approach to decimals, in which there is ignorance of the fact that students see decimals everywhere, from money to measurement, yet the discussion of decimals is made dependent on fractions that are far more difficult and less in the world of students. - Infinity, infinite decimals, limits.
The word “infinite” never appears in the Common Core even once. Amazing. Are we not to teach students that there are infinitely many integers? What about the end behavior of functions? There is no discussion of infinite decimals, so what are students to learn about π? The only mention of limits is in connection with informal arguments about the areas and volumes of curved figures. The phrase “infinitely many” appears twice, both times to describe how many solutions there are to inequalities. First-graders can understand that there are infinitely many integers because they can see that if there is a largest number, then there is a number one larger. That was part of the curriculum in the PBS program 3-2-1 Contact decades ago. - Deductive systems (what is deduction, roles of postulates, undefined terms, definitions, theorems)
There is nothing about mathematical systems. It is like teaching chemistry without the periodic table, or biology without the classification of living things, or history without a time line. In high school, students should see that mathematical truth rests on careful definitions of terms and deduction from commonly agree-upon postulates.
Changes Needed in the Testing Program
The problems with the Common Core could be ignored if there were no testing program based on them. Then the weaknesses in the CCSSM would be somewhat like the weaknesses we can find in almost any program available. But the testing program is so flawed that it is going to be a disaster. There are problems with the questions that are being proposed and the number of tests, and these problems are compounded by what we already see will be the interpretation of the disastrous results that are bound to occur.
Changes needed in the questions
What kinds of questions are students being asked to solve? On the PARCC website, there are now 34 sample tasks. Here is a sample task for high school that is identified as “seeing structure in a quadratic equation”.
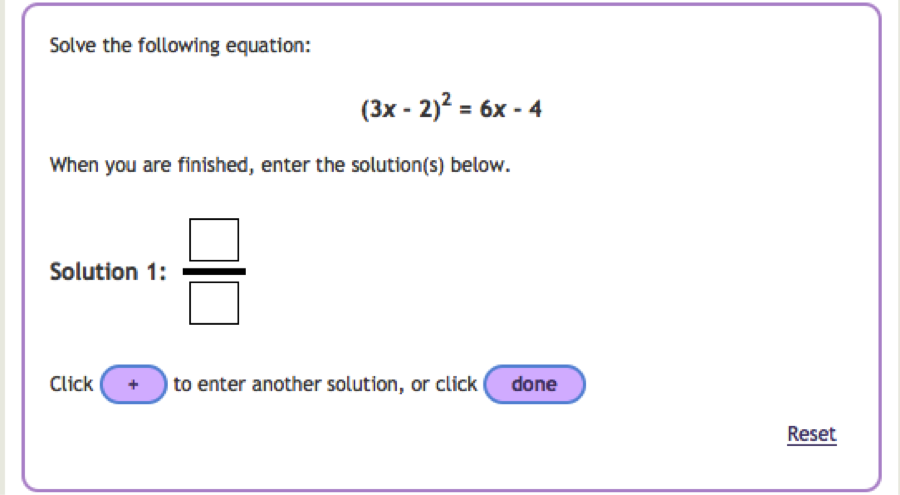
Suppose I am a student and I see the structure. The right side is twice 3x – 2. Ah! I can divide both sides by 3x – 2. I get 3x – 2 = 2, so 3x = 4, and x = 4/3. I substitute back in the original equation and it works.
The only problem is that I have not done the question correctly. I have forgotten that I cannot divide by 3x – 2 when 3x – 2 = 0. And when 3x – 2 = 0, x = 2/3, and that is a second solution to the equation. I have lost a solution by dividing both sides by 3x – 2.
If I don’t see the structure, what do I do? I expand the left side to obtain 9x2 – 12x + 4 = 6x – 4, I add -6x and 4 to both sides to get 9x2 + 6x + 8 = 0, I use the quadratic formula and I get two solutions, 2/3 and 4/3. So if I do not see the structure, I might be more likely to get the correct answer.
The addition and loss of solutions to equations is typical precalculus content, 12th-grade mathematics. This question is what you discuss when you are teaching about extraneous solutions to equations. It is the kind of question found on math contests because it is so tricky. It does not belong on a test over a “common core”.
Questions like these survive on tests because a percentage of students between 10% and 90% get them correct, so they are viewed as not too difficult or too easy, and because the tendency is for the top-scoring students to get them correct more often than the bottom-scoring students. Sure, that is how these students got to be top-scoring! But the face validity of the question and its relationship to the curriculum need to be given at least as much weight in viewing the item.
A second sample question on the PARCC website is the following 6th grade item, called “Slider Ruler”.


Do you think the item is a little tricky? There is evidence that it is and we do not have to pretest the item to know this, because this item is directly related to a famous problem from the research of John Clement, Jack Lochhead, and George Monk in 1980. Here is their problem:
Write an equation for the following statement: “There are six times as many professors as students at this university.” Use S for the number of students and P for the number of professors.
On a written test with 150 calculus-level students, 37 percent missed this problem, and two-thirds of the errors took the form of a reversal of variables such as 6S = P. In a sample of 47 nonscience majors taking college algebra, the error rate was 57 percent (Clement, Lochhead, and Monk (1981).
The problem was tricky for college mathematics students and here it is, a sample problem over the common core for 6th-graders. Furthermore, it turns out that it was also reported that “Difficulty in solving this and similar problems is highly resistant to remediation.” (Rosnick, P. and Clement, J. (1980), as reported in Fisher (1988).
Moreover, the PARCC sample item presents even worse difficulties than the professors and student example. Because 1 inch = 2.54 centimeters, it is natural to think that I = 2.54c. A student has to wonder what answer the test-takers wanted. Finally, look again at the choices. Do you need a slider to answer this question? Does the slider help or make things more confusing? Certainly it will cause students to spend more time on the item, and it may cause students to wonder whether they are supposed to learn something new from the slider and thus possibly change the answer they might get without the slider. Regardless, this kind of question is totally inappropriate, and it is given as a sample of what to expect from PARCC.
The questions on a test of the common core should be core questions, not tricks or contest problems. The questions on a test of the common core should be appropriate to the understandings that a student should be expected to have at the grade at which the test is taken, not the understandings that a typical student acquires only after years of experience with the content.
I know that some of the more recent PARCC items have been passed by people for their comments because I was one of the people in a group who commented. But our comments were largely limited to editing; any thoughts that the entire idea behind a question was poor were ignored.
The Smarter Balanced website shows 27 sample items. There are difficulties with a few of them, but overall I think they may be better than those of PARCC. However, I cannot judge their feasibility because I could not find the particular grade level at which any item was to be given.
The questions on the Common Core tests need rigorous review and pre-testing with appropriate populations before they appear. For ten years I was a member of the NAEP Mathematics Test Committee that edited the NAEP tests. I considered myself very experienced and knowledgeable not only about the curriculum but about setting test items for students. It was amazing to me to see, at every meeting that this NAEP committee had, how many people found problems that I did not discern, problems with question wordings, with bias of one kind or another, with interpretations of how students could get the right answer for the wrong reason or the wrong answer even though they knew the content. Very few questions survived intact. I think very many of the sample items I have viewed on the websites would have been edited in some way by the committee.
Changes needed in how people interpret scores on the tests
Kentucky was the first state to adopt the common core, and in 2012 became the first state to give an assessment based on the common core curriculum it had adopted. In grades 3-5, the percent of proficient-or-higher students dropped from 70% to 47%; in grades 6-8, the drop was from 65% to 41%. In high school, the drop was much less, from 46% to 40%.
What was the reaction of the Kentucky education commissioner, Terry Holliday? He is quoted in Education Week (7 Nov 2012) as saying “We knew the scores were going to drop, but this is the right thing for our kids, our schools.” In my opinion, he is happy the scores dropped because it justifies the common core and the common core testing. In fact, if the scores increased, he probably would have complained that the test was too easy.
The Education Week article writer interviewed Douglas McRae, a retired assessment designer who helped build California’s testing system. McRae pointed out what many of us know. When new tests are introduced, states can expect scores to fall in most cases. “When you change the measure, change the tests, then you interrupt the continuity of trend data over time. That’s the fundamental thing that happens,” he said.
This quote is disturbing on two fronts. First is the expectation that scores will fall. If it’s a new test, what will they fall from? In Kentucky it was the percent of students scoring proficient or higher, but the earlier test was completely different from the Common Core test. Would students now score lower on the old test also? We have no idea.
It is important to keep track of how students would do on earlier measures of performance, because our successes have been lost in the Common Core rhetoric. Although it is perhaps the major weakness of our educational system that the mean scores of Blacks and Hispanics on National Assessment at grades 4 and 8 are about two years behind the mean scores of Asians and Whites, the mean scores for all groups have increased significantly since the 1970s on the long-term longitudinal study and since 1990 on the short-term study.
Table 2. Changes in indicators of mathematics performance of U.S. students, 1990-2013.
| Indicator | Before NCTM Standards | Most Recent Result | ||
|---|---|---|---|---|
| NAEP 4th grade average math score | 1990 | 213 | 2013 | 242 |
| NAEP 8th grade average math score | 1990 | 263 | 2013 | 285 |
| SAT-M mean score of seniors | 1990 | 501 | 2013 | 514 |
| ACT-M mean score | 1990 | 19.9 | 2013 | 20.9 |
| Number of ACT test-takers | 1990 | 817,000 | 2013 | 1,799,000 |
Sources: NAEP, NCES, College Board, and ACT, Inc.
Table 2 shows what has happened since 1990 on the main national indicators of mathematics performance. An increase in 14 points on the NAEP is considered about equivalent to a year’s study. Since 1990, we have had an overall increase on NAEP of about the equivalent of two grades at 4th grade and about 1.5 grades at 8th grade. The mean score on the SATs increased from 501 in 1990 to 514 in 2013, having stagnated since the introduction of a third test. The mean score on the ACTs increased from 19.9 to 20.9 from 1990 to 2013 despite more than a doubling of the number of students taking the ACTs. What will happen to those trends? It is important that we keep long-term trend data so that we can know how the Common Core is faring on traditional measures.
If there becomes some move to change these tests significantly due to the standards, and it seems that there will be with the SAT and ACT, a revised standards should call for their reinstatement so that we can see whether the Common Core has raised achievement.
Also lost in the Common Core rhetoric is the extraordinary success of a nationwide program that involves our best students, AP calculus. I was in Japan last year speaking to Japanese mathematics educators who are often in the United States and showed the following data. The response of one of those educators was “I had no idea. We thought there were no good students in the United States!” And so excuse my departure from the Common Core to talk about this success.
About 18% of high school graduates took calculus in 2009, up from 14% just five years earlier and triple the 6% of graduates who took calculus in 1982. The number of students who took the Advanced Placement calculus tests offered by the College Board in 2012 is 13 times what it was in 1980 and had doubled even since 2000. Because the number of students who currently take calculus in high school is about double the number who take the exams, far more students are now taking a full-year course in calculus in high school than are taking calculus in college.
With so many more students taking the test, you would think that the percent of students obtaining the highest grades, or the percentage passing, would decrease. But through this period, those percentages have increased both on the AB and BC forms of the test. As you know, the best colleges require a 4 or a 5 on the AB test or a 3, 4, or 5 on the BC test to give credit. Table 3 shows that since 1999, the number of AB test takers has a little bit more than doubled, and the number of BC test-takers has a little more than tripled. And yet the number of top scores has been multiplied by over 3.5 for the AB test, and it has been multiplied by over 4 on the BC test. That is, contrary to what usually happens when a population taking a difficult test increases, today’s much greater numbers of students are scoring higher than their more select counterparts of not long ago. In 1999, 38% of AB test-takers scored 4 or 5; in 2012, 42% did. In 1999, 79% of BC test-takers scored 3, 4, or 5; in 2012 82% did. I could not find the corresponding raw data for 2013, but the percents scoring 4 or 5 on the AB and 3, 4, or 5 on the BC were the same as in 2012.
Table 3: Successful AP Calculus Students, 1999-2012
| Year | 4 on AB | 5 on AB | 3 on BC | 4 on BC | 5 on BC | Total |
|---|---|---|---|---|---|---|
| 1999 | 27,936 | 19,763 | 6,797 | 5,566 | 11,616 | 71,678 |
| 2001 | 33,177 | 22,480 | 8,722 | 6,432 | 14,505 | 85,316 |
| 2004 | 34,854 | 35,755 | 10,496 | 9,416 | 19,971 | 110,492 |
| 2006 | 40,353 | 43,925 | 11,537 | 11,524 | 24,561 | 131,900 |
| 2008 | 45,470 | 46,363 | 13,117 | 11,692 | 28,561 | 145,203 |
| 2010 | 40,418 | 52,148 | 14,218 | 12,164 | 39,012 | 157,960 |
| 2012 | 45,523 | 67,394 | 14,957 | 15,231 | 47,553 | 190,658 |
Source: AP Reports to the Nation, various years, other College Board reports
The Common Core does not mention calculus in high school. Its rhetoric is: “The high school standards specify the mathematics that all students should study in order to be college and career ready. Additional mathematics that students should learn in order to take advanced courses such as calculus, advanced statistics, or discrete mathematics is indicated by (+)…” This is understandable in a set of standards for all students. But it is not clear what will happen with this population in a Common Core era.
Before the Common Core there was not much new content introduced in Grade 8, so a strengthened Grade 7 followed by teaching algebra or an integrated Grade 9 course in Grade 8 was much easier to cover. Any weaknesses in background would be covered by the higher expectations in the high school courses the best students take. I worry that putting all the pressure on the middle school, coupled with a greater number of tests that can serve as filters, will decrease the number of students who finish what has to be considered perhaps the greatest success story of the recent past in school mathematics education.
Reduce the number of tests.
For well over a half-century, many schools and school districts have given elementary school students standardized tests every year. These tests have been used primarily in two ways: they tell teachers who are the better-prepared students and who are the lesser-prepared students, and they are often used as one indicator to sort students into advanced, regular, or remedial classes. Although most of these tests provide detailed information about strengths and weaknesses a student has in various areas of mathematics, I think that in most schools that that detailed information is not often used.
Should we expect more from PARCC or Smarter Balanced? I doubt that more is possible. Because there are tests in language arts and mathematics, the two most important subjects in the elementary school, and because students who score poorly in one of these areas tend to score poorly in the other, I foresee huge numbers of students being held back whereas without the tests they would remain with their peers. The research evidence is that holding students back does not increase performance, so we will have more students who are older than their classmates and these students will be more likely to drop out.
No country in the world gives high stakes tests every year. Yet these Common Core tests will be high-stakes, with schools ranked by their results and, in some places, teachers ranked as well.
If those who conceptualized the Common Core really wanted to emulate Singapore and some other nations of East Asia, then they would have had only two tests, one at 6th grade and one at 9th. A second edition of the Common Core should be honest with the mathematics education community. It should indicate which ideas that are being proposed are untested and which ideas constitute a policy different from those of other countries with whom we are compared. But we have to be diligent. Some people in high positions are test-crazy.
You may not agree with my opinions, but I hope that I have at least spurred you to think that we should be starting now to think about what a revision of the Common Core would look like.
References
All references to the Common Core in this paper are from the Common State Standards Initiative, Common Core State Standards for Mathematics, downloaded by this author 12 Jan 2011.
American Statistical Association (2007). Guidelines for Assessment and Instruction in Statistics Education (GAISE). Available at www.amstat.org/education/gaise/index.cfm.
Clement, John, Jack Lochhead, and George S. Monk (April 1981). Translation Difficulties in Learning Mathematics. American Mathematical Monthly 88(4), 286-290.
Daro, Phil (2012). Remarks made in a speech at the Metropolitan Mathematics Club of Chicago, 9 Nov 2012.
Fuson, Karen C., and Sybilla Beckmann (2012/2013). Standard Algorithms in the Common Core State Standards. NCSM Journal, Fall/Winter 2012-2013, pp. 14-30.
PARCC (Partnership for Assessment of Readiness for College and Careers) (2014). parcc.pearson.com/practice-tests/math/
Ravitch, Diane (2014). Diane Ravitch’s blog: A site to discuss better education for all. www.dianeravitch.net/category/common-core/.
Rosnick, Peter. and John Clement (1980). “Learning without understanding: The effect of tutoring strategies on algebra misconceptions.” Journal of Mathematical Behavior 3(1), 3-28, as reported in Kathleen M. Fisher (1988). The Students-and-Professors Problem Revisited. JRME 19(3), 260-262.
Schmidt, William H., Curtis C. McKnight, and Senta A. Raizen (1996). A Splintered Vision: An Investigation of U.S. Science and Mathematics Education. Dordrecht: Kluwer Academic Publishers.
U.S. Department of Education (2013). Education Department Launches 2013 Investing in Innovation Competition. Retrieved from www.ed.gov/news/press-releases/education-department-launches-2013-investing-innovation-competition.
Contact
UCSMP
1427 East 60th Street
Chicago, IL 60637
T: 773-702-1130
F: 773-834-4665
ucsmp@uchicago.edu