UCSMP Geometry
Main goal: The main goal of UCSMP Geometry is to provide students with a clear understanding of two-dimensional and three-dimensional figures and the relationships among them.
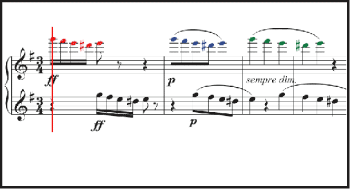
Main theme I: Geometry applies to all figures and all physical objects, not just lines, angles, triangles, other polygons, and circles. Transformations allow general definitions of congruence, similarity, and symmetry and make it easy to connect the abstract notions of geometry with the figures on a page and the real world. Special lessons are devoted to aspects of geometry in art, architecture, sports, and music.
Main theme II: The course operates within a coherent mathematical system. Starting from the assumed properties of points, lines, and angles, the book systematically develops the general properties of congruence and similarity and applies them to deduce specific properties of the common figures. Proofs are gradually introduced as students learn how to write 2-column and paragraph proofs. Indirect proofs and coordinate geometry proofs are also covered.
Main theme III: The study of perimeter, area, surface area, and volume in two-dimensional and three-dimensional figures, both real and abstract, is covered extensively. Special emphasis is given to the study of three-dimensional figures and to showing how area and volume formulas are related to one another.
Main theme IV: The integration of algebra and geometry starts in the first chapter with the introduction of the coordinate geometry of lines. This coincides with the belief that students will have a better grasp on geometric concepts when they are taught in relation to algebraic skills they have already learned. Transformations provide practice with function notation and composites of functions. Students learn to use algebra to derive properties of figures by using a coordinate plane to place a figure on.
Comparison between this and earlier editions: In this edition, activities with dynamic geometry systems run throughout the book. New lessons and problems emphasize connections with the arts. Question sets in most lessons have been revamped.
Some distinctive lessons: The need for definitions (2-1); Transformations and music (4-9); Frieze patterns (6-9); Can there be giants? (12-5); The isoperimetric inequality (14-8).
Music Animations Applet Demonstrating Transformations
Contact
UCSMP
1427 East 60th Street
Chicago, IL 60637
T: 773-702-1130
F: 773-834-4665
ucsmp@uchicago.edu